|
|
The Australian Journal of Mathematical Analysis and Applications
ISSN 1449-5910 |
|
||||||||||||
|
|
||||||||||||
Paper's Title:
The Superstability of the Pexider Type Trigonometric Functional Equation
Author(s):
Gwang Hui Kim and Young Whan Lee
Department of Mathematics, Kangnam
University Yongin, Gyeonggi, 446-702, Korea.
ghkim@kangnam.ac.kr
Department of Computer and Information Security
Daejeon University, Daejeon 300-716, Korea.
ywlee@dju.ac.kr
Abstract:
The aim of this paper is to investigate the stability
problem for
the Pexider type (hyperbolic) trigonometric functional equation
f(x+y)+f(x+σy)=λg(x)h(y) under the conditions :
|f(x+y)+f(x+σy)- λg(x)h(y)|≤φ(x),
φ(y), and min {φ(x), φ (y)}.
As a consequence, we have generalized the results of stability for
the cosine(d'Alembert), sine, and the Wilson functional equations by J.
Baker, P. Găvruta, R. Badora and R. Ger, Pl.~Kannappan, and G.
H. Kim
Paper's Title:
A Caratheodory's Approximate Solutions of Stochastic Differential Equations Under the Hölder Condition
Author(s):
Bo-Kyeong Kim and Young-Ho Kim
Department of Mathematics,
Changwon National University,
Changwon, Gyeongsangnam-do 51140,
Korea.
E-mail: claire9576@naver.com
yhkim@changwon.ac.kr
Abstract:
In this paper, based on the theorem of the uniqueness of the solution of the stochastic differential equation, the convergence possibility of the Caratheodory's approximate solution was studied by approximating the unique solution. To obtain this convergence theorem, we used a Hölder condition and a weakened linear growth condition. Furthermore, The auxiliary theorems for the existence and continuity of the Caratheodory's approximate solution were investigated as a prerequisite.
Paper's Title:
Derivation of the Existence Theorem of the Solution of the Stochastic Functional Differential Equation Using Conditions Given Partial Weights
Author(s):
Young-Ho Kim
Department of Mathematics,
Changwon National University
Changwon, Gyeongsangnam 51140,
Korea.
E-mail:
yhkim@changwon.ac.kr
Abstract:
The main purpose of this note was to demonstrate the solution existence theorem for stochastic functional differential equations under sufficient conditions. As an alternative to the stochastic process theory of the stochastic functional differential equations, we impose a partial weighting condition and a weakened linear growth condition. We first show that the condition guarantees existence and uniqueness and then show some exponential estimates for the solution.
Paper's Title:
A Stability of the G-type Functional Equation
Author(s):
Gwang Hui Kim
Department of Mathematics, Kangnam University
Suwon 449-702, Korea.
ghkim@kangnam.ac.kr
Abstract:
We will investigate the stability in the sense of G
Paper's Title:
Evaluation of a New Class of Double Integrals Involving Generalized Hypergeometric Function
4F3
Author(s):
Joohyung Kim, Insuk Kim and Harsh V. Harsh
Department of Mathematics Education,
Wonkwang University, Iksan, 570-749,
Korea.
E-mail: joohyung@wku.ac.kr
Department of Mathematics Education,
Wonkwang University, Iksan, 570-749,
Korea.
E-mail: iki@wku.ac.kr
Department of Mathematics, Amity School
of Eng. and Tech.,
Amity University Rajasthan
NH-11C, Jaipur-303002, Rajasthan,
India.
E-mail: harshvardhanharsh@gmail.com
Abstract:
Very recently, Kim evaluated some double integrals involving a generalized hypergeometric function 3F2 with the help of generalization of Edwards's well-known double integral due to Kim, et al. and generalized classical Watson's summation theorem obtained earlier by Lavoie, et al. In this research paper we evaluate one hundred double integrals involving generalized hypergeometric function 4F3 in the form of four master formulas (25 each) viz. in the most general form for any integer. Some interesting results have also be obtained as special cases of our main findings.
Paper's Title:
Two Further Methods for Deriving Four Results Contiguous to Kummer's Second Theorem
Author(s):
I. Kim and J. Kim
Department of Mathematics Education,
Wonkwang University,
Iksan, 570-749,
Korea.
E-mail: iki@wku.ac.kr
Department of Mathematics Education,
Wonkwang University,
Iksan, 570-749,
Korea.
E-mail: joohyung@wku.ac.kr
Abstract:
In the theory of generalized hypergeometric function, transformation and summation formulas play a key role. In particular, in one of the Kummer's transformation formulas, Kim, et al. in 2012, have obtained ten contiguous results in the form of a single result with the help of generalization of Gauss's second summation theorem obtained earlier by Lavoie, et al.. In this paper, we aim at presenting four of such results by the technique of contiguous function relations and integral method developed by MacRobert.
Paper's Title:
An Existence of the Solution to Neutral Stochastic Functional Differential Equations Under the Holder Condition
Author(s):
Young-Ho Kim
Department of Mathematics,
Changwon National University,
Changwon, Gyeongsangnam-do 51140,
Korea.
E-mail: iyhkim@changwon.ac.kr
Abstract:
In this paper, we show the existence and uniqueness of solution of the neutral stochastic functional differential equations under weakened H\"{o}lder condition, a weakened linear growth condition, and a contractive condition. Furthermore, in order to obtain the existence of a solution to the equation we used the Picard sequence.
Paper's Title:
Viability
Theory And Differential Lanchester Type Models For Combat.
Differential Systems.
Author(s):
G. Isac and A. Gosselin
Department Of
Mathematics, Royal Military College Of Canada,
P.O. Box 17000, Stn Forces, Kingston,
Ontario, Canada K7k 7b4
isac-g@rmc.ca
gosselin-a@rmc.ca
URL:
http://www.rmc.ca/academic/math_cs/isac/index_e.html
URL:
http://www.rmc.ca/academic/math_cs/gosselin/index_e.html
Abstract:
In 1914, F.W. Lanchester proposed several mathematical models based on differential equations to describe combat situations [34]. Since then, his work has been extensively modified to represent a variety of competitions including entire wars. Differential Lanchester type models have been studied from many angles by many authors in hundreds of papers and reports. Lanchester type models are used in the planning of optimal strategies, supply and tactics. In this paper, we will show how these models can be studied from a viability theory stand point. We will introduce the notion of winning cone and show that it is a viable cone for these models. In the last part of our paper we will use the viability theory of differential equations to study Lanchester type models from the optimal theory point of view.
Paper's Title:
On an extension of Edwards's double integral with applications
Author(s):
I. Kim, S. Jun, Y. Vyas and A. K. Rathie
Department of Mathematics Education,
Wonkwang University,
Iksan, 570-749,
Republic of Korea.
General Education Institute,
Konkuk University,
Chungju 380-701,
Republic of Korea.
Department of Mathematics, School of
Engineering,
Sir Padampat Singhania University,
Bhatewar, Udaipur, 313601, Rajasthan State,
India.
Department of Mathematics,
Vedant College of Engineering and Technology,
(Rajasthan Technical University),
Bundi-323021, Rajasthan,
India.
E-mail: iki@wku.ac.kr
sjun@kku.ac.kr
yashoverdhan.vyas@spsu.ac.in
arjunkumarrathie@gmail.com
Abstract:
The aim of this note is to provide an extension of the well known and useful Edwards's double integral. As an application, new class of twelve double integrals involving hypergeometric function have been evaluated in terms of gamma function. The results are established with the help of classical summation theorems for the series 3F2 due to Watson, Dixon and Whipple. Several new and interesting integrals have also been obtained from our main findings.
Paper's Title:
Fractional exp(-φ(ξ))- Expansion Method and its Application to Space--Time Nonlinear Fractional Equations
Author(s):
A. A. Moussa and L. A. Alhakim
Department of Management Information
System and Production Management,
College of Business and Economics, Qassim University,
P.O. BOX 6666, Buraidah: 51452,
Saudi Arabia.
E-mail: Alaamath81@gmail.com
URL:
https://scholar.google.com/citations?user=ccztZdsAAAAJ&hl=ar
Department of Management Information
System and Production Management,
College of Business and Economics, Qassim University,
P.O. BOX 6666, Buraidah: 51452,
Saudi Arabia.
E-mail: Lama2736@gmail.com
URL:
https://scholar.google.com/citations?user=OSiSh1AAAAAJ&hl=ar
Abstract:
In this paper, we mainly suggest a new method that depends on the fractional derivative proposed by Katugampola for solving nonlinear fractional partial differential equations. Using this method, we obtained numerous useful and surprising solutions for the space--time fractional nonlinear Whitham--Broer--Kaup equations and space--time fractional generalized nonlinear Hirota--Satsuma coupled KdV equations. The solutions obtained varied between hyperbolic, trigonometric, and rational functions, and we hope those interested in the real-life applications of the previous two equations will find this approach useful.
Paper's Title:
A Review on Minimally Supported Frequency Wavelets
Author(s):
K Pallavi1, M C Lineesh1, A Noufal2
1
Department of Mathematics,2Department of Mathematics,
Cochin University of Science and Technology,
Kerala 682022,
India.
E-mail: noufal@cusat.ac.in
Abstract:
This paper provides a review on Minimally Supported Frequency (MSF) wavelets that includes the construction and characterization of MSF wavelets. The characterization of MSF wavelets induced from an MRA is discussed and the nature of the low-pass filter associated with it is explained. The concept of wavelet set and dimension function is introduced to study this class of wavelets. Along with MSF wavelets, s-elementary wavelets and unimodular wavelets are also considered due to the similarity in definitions. Examples and illustrations are provided for more clarity.
Paper's Title:
Approximately Dual p-Approximate Schauder Frames
Author(s):
K. Mahesh Krishna and P. Sam Johnson
Stat-Math Unit, Indian Statistical
Institute, Bangalore Centre,
Karnataka 560 059
India.
Department of Mathematical and
Computational Sciences,
National Institute of Technology Karnataka (NITK),
Surathkal, Mangaluru 575 025,
India.
E-mail: kmaheshak@gmail.com
sam@nitk.edu.in
Abstract:
Approximately dual frame in Hilbert spaces was introduced by Christensen and Laugesen to overcome difficulties in constructing dual frames for a given Hilbert space frame. It becomes even more difficult in Banach spaces to construct duals. For this purpose, we introduce approximately dual frames for a class of approximate Schauder frames for Banach spaces and develop basic theory. Approximate dual for this subclass is completely characterized and its perturbation is also studied.
Paper's Title:
ℵ0 Algebra and its Novel Application in Edge Detection
Author(s):
G. E. Chatzarakis1, S. Dickson2, S. Padmasekaran2, S. L. Panetsos1, and J. Ravi3
1Electrical
and Electronic Engineering Educators,
School of Pedagogical and Technological Education (ASPETE),
Marousi 15122, Athens,
Greece.
E-mail: geaxatz@otenet.gr,
spanetsos@aspete.gr
2Mathematics,
Periyar University,
Periyar Palkalai Nagar, Salem, 636011, Tamilnadu,
India.
E-mail: dix.bern@gmail.com,
padmasekarans@periyaruniversity.ac.in
3Department
of Mathematics, Amity University,
Bengaluru, Karnataka,
India.
E-mail:
jravistat@gmail.com
Abstract:
In this paper a new type of ℵ0-algebra has been defined. With its help, the fuzzy cross subalgebra and the fuzzy η-relation on the ℵ0-algebra are introduced and their respective properties are derived. Moreover, the fuzzy cross ℵ0-ideal of the ℵ0-algebra is defined with some theorems and intuitionistic fuzzy ℵ0-ideals of the ℵ0-algebra are introduced. This fuzzy algebra concept is applied in image processing to detect edges. This ℵ0-algebra is a novelty in the field of research.
Paper's Title:
On the Hohov Convolution Of The Class Sp(α,β)
Author(s):
T. N. Shanmugam and S. Sivasubramanian
Department of Mathematics,
Anna University,
Chennai 600025,
Tamilnadu, India.
shan@annauniv.edu
Department of Mathematics,
Easwari Engineering College,
Chennai-600089,
Tamilnadu, India,
sivasaisastha@rediffmail.com
Abstract:
Let F(a,b;c;z) be the Gaussian hypergeometric function and Ia,b;c(f)=zF(a,b;c;z)*f(z) be the Hohlov operator defined on the class A of all normalized analytic functions. We determine conditions on the parameters a,b,c such that Ia,b;c(f) will be in the class of parabolic starlike functions Sp(α,β). Our results extend several earlier results.
Paper's Title:
Komatu Integral Transforms of Analytic Functions Subordinate to Convex Functions
Author(s):
T. N. Shanmugam and C. Ramachandran
Department of Mathematics, College of Engineering,
Anna University, Chennai-600 025, Tamilnadu,
India
shan@annauniv.edu
Department of Mathematics, College of Engineering,
Anna University, Chennai-600 025, Tamilnadu,
India
crjsp2004@yahoo.com
Abstract:
In this paper, we consider the class A of the functions f(z) of the form
which are analytic in an open disk
has some property. Certain inclusion and the closure properties like convolution with convex univalent function etc. are studied.

 and study certain subclass of the class A, for which
and study certain subclass of the class A, for which
Paper's Title:
Approximation of an AQCQ-Functional Equation and its Applications
Author(s):
Choonkil Park and Jung Rye Lee
Department of Mathematics,
Research Institute for Natural Sciences,
Hanyang University, Seoul 133-791,
Korea;
Department of Mathematics,
Daejin University,
Kyeonggi 487-711,
Korea
baak@hanyang.ac.kr
jrlee@daejin.ac.kr
Abstract:
This paper is a survey on the generalized Hyers-Ulam stability of an AQCQ-functional equation in several spaces. Its content is divided into the following sections:
1. Introduction and preliminaries.
2. Generalized Hyers-Ulam stability of an AQCQ-functional equation in Banach spaces: direct method.
3. Generalized Hyers-Ulam stability of an AQCQ-functional equation in Banach spaces: fixed point method.
4. Generalized Hyers-Ulam stability of an AQCQ-functional equation in random Banach spaces: direct method.
5. Generalized Hyers-Ulam stability of an AQCQ-functional equation in random Banach spaces: fixed point method.
6. Generalized Hyers-Ulam stability of an AQCQ-functional equation in non-Archi-medean Banach spaces: direct method.
7. Generalized Hyers-Ulam stability of an AQCQ-functional equation in non-Archi-medean Banach spaces: fixed point method.
Paper's Title:
On a New Class of Eulerian's Type Integrals Involving Generalized Hypergeometric Functions
Author(s):
Sungtae Jun, Insuk Kim and Arjun K. Rathie
General Education Institute,
Konkuk University, Chungju 380-701,
Republic of Korea.
Department of Mathematics Education,
Wonkwang University, Iksan, 570-749,
Republic of Korea.
Department of Mathematics,
Vedant College of Engineering and Technology (Rajasthan Technical University),
Bundi-323021, Rajasthan,
India.
E-mail: sjun@kku.ac.kr, iki@wku.ac.kr, arjunkumarrathie@gmail.com
Abstract:
Very recently Masjed-Jamei and Koepf established interesting and useful generalizations of various classical summation theorems for the 2F1, 3F2, 4F3, 5F4 and 6F5 generalized hypergeometric series. The main aim of this paper is to establish eleven Eulerian's type integrals involving generalized hypergeometric functions by employing these theorems. Several special cases have also been given.
Paper's Title:
A New Proof of Classical Watson's Summation Theorem for the Series 3F2(1)
Author(s):
Insuk Kim, Arjun K. Rathie
Department of Mathematics Education,
Wonkwang University,
Iksan, 570-749,
Korea.
E-mail: iki@wku.ac.kr
Department of Mathematics,
Vedant College of Engineering and Technology (Rajasthan Technical University),
Bundi, Rajasthan,
India.
E-mail:
arjunkumarrathie@gmail.com
Abstract:
The aim of this short research note is to provide a new proof of classical Watson's summation theorem for the series 3F2(1). The theorem is obtained by evaluating an infinite integral and making use of classical Gauss's first and second summation theorems for the series 2F1.
Paper's Title:
Norm Estimates for the Difference between Bochner’s Integral and the Convex Combination of Function’s Values
Author(s):
P. Cerone, Y.J. Cho, S.S. Dragomir, J.K. Kim, and S.S. Kim
School of
Computer Science and Mathematics,
Victoria University of Technology,
Po Box 14428, Mcmc 8001, Victoria, Australia.
pietro.cerone@vu.edu.au
URL:
http://rgmia.vu.edu.au/cerone/index.html
Department of
Mathematics Education, College of Education,
Gyeongsang National University, Chinju 660-701, Korea
yjcho@nongae.gsnu.ac.kr
School of
Computer Science and Mathematics,
Victoria University of Technology,
Po Box 14428, Mcmc 8001, Victoria, Australia.
sever.dragomir@vu.edu.au
URL:
http://rgmia.vu.edu.au/SSDragomirWeb.html
Department of
Mathematics, Kyungnam University,
Masan,, Kyungnam 631-701, Korea
jongkyuk@kyungnam.ac.kr
Department of
Mathematics, Dongeui University,
Pusan 614-714, Korea
sskim@dongeui.ac.kr
Abstract:
Norm estimates are developed between the Bochner integral of a vector-valued function in Banach spaces having the Radon-Nikodym property and the convex combination of function values taken on a division of the interval [a, b].
Paper's Title:
Algebraic Approach to the Fractional Derivatives
Author(s):
Kostadin Trenčevski and Živorad Tomovski
Institute of Mathematics,
St. Cyril and Methodius University,
P.O. Box 162, 1000 Skopje,
Macedonia
kostatre@iunona.pmf.ukim.edu.mk
Abstract:
In this paper we introduce an alternative definition of the fractional derivatives and also a characteristic class of so called ideal functions, which admit arbitrary fractional derivatives (also integrals). Further some ideal functions are found, which lead to representations of the Bernoulli and Euler numbers Bk and Ek for any real number k, via fractional derivatives of some functions at x=0.
Paper's Title:
Stability of a Mixed Additive, Quadratic and Cubic Functional Equation In Quasi-Banach Spaces
Author(s):
A. Najati and F. Moradlou
Department of Mathematics, Faculty of Sciences,
University of Mohaghegh Ardabili, Ardabil,
Iran
a.nejati@yahoo.com
Faculty of Mathematical Sciences,
University of Tabriz, Tabriz,
Iran
moradlou@tabrizu.ac.ir
Abstract:
In this paper we establish the general solution of a mixed additive,
quadratic and cubic functional equation and investigate the
Hyers--Ulam--Rassias stability of this equation in quasi-Banach
spaces. The concept of Hyers-Ulam-Rassias stability originated from
Th. M. Rassias' stability theorem that appeared in his paper: On the
stability of the linear mapping in Banach spaces, Proc. Amer. Math.
Soc. 72 (1978), 297--300.
Paper's Title:
On the Generalized Stability and
Asymptotic Behavior of Quadratic Mappings
Author(s):
Hark-Mahn Kim, Sang-Baek Lee and Eunyoung Son
Department of Mathematics
Chungnam National University
Daejeon,
305-764,
Republic of Korea
hmkim@cnu.ac.kr
Abstract:
We extend the stability of quadratic mappings to the stability of general quadratic mappings with several variables, and then obtain an improved asymptotic property of quadratic mappings on restricted domains.
Paper's Title:
Shape Diagrams for 2D Compact Sets - Part III: Convexity
Discrimination for Analytic and Discretized Simply Connected Sets.
Author(s):
S. Rivollier, J. Debayle and J.-C. Pinoli
Ecole Nationale Supérieure des Mines de Saint-Etienne,
CIS - LPMG, UMR CNRS
5148,
158 cours Fauriel,
42023 Saint-Etienne Cedex 2, France.
rivollier@emse.fr;
debayle@emse.fr; pinoli@emse.fr
Abstract:
Shape diagrams are representations in the Euclidean plane introduced to study 3-dimensional and 2-dimensional compact convex sets. However, they can also been applied to more general compact sets than compact convex sets. A compact set is represented by a point within a shape diagram whose coordinates are morphometrical functionals defined as normalized ratios of geometrical functionals. Classically, the geometrical functionals are the area, the perimeter, the radii of the inscribed and circumscribed circles, and the minimum and maximum Feret diameters. They allow twenty-two shape diagrams to be built. Starting from these six classical geometrical functionals, a detailed comparative study has been performed in order to analyze the representation relevance and discrimination power of these twenty-two shape diagrams. The two first parts of this study are published in previous papers 8,9. They focus on analytic compact convex sets and analytic simply connected compact sets, respectively. The purpose of this paper is to present the third part, by focusing on the convexity discrimination for analytic and discretized simply connected compact sets..
Paper's Title:
Inclusion Properties of a Certain Subclass of Strongly Close-To-Convex Functions
Author(s):
S. M. Khairnar and M. More
Department of Mathematics,
Maharashtra Academy of Engineerring,
Alandi -412 105, Pune, Maharashtra,
INDIA.
smkhairnar2007@gmail.com,
meenamores@gmail.com.
Abstract:
The purpose of this paper is to derive some inclusion and argument properties of a new subclass of strongly close-to-convex functions in the open unit disc. We have considered an integral operator defined by convolution involving hypergeometric function in the subclass definition. The subclass also extends to the class of α-spirallike functions of complex order.
Paper's Title:
Stability Problems for Generalized Additive Mappings and Euler-Lagrange Type Mappings
Author(s):
M. Todoroki, K. Kumahara, T. Miura and S.-E. Takahasi
The Open University of Japan,
Chiba, 261-8586,
Japan
tomamiyu3232@sky.sannet.ne.jp
kumahara@ouj.ac.jp
Yamagata University,
Yonezawa 992-8510,
Japan
miura@yz.yamagata-u.ac.jp
Toho University, Yamagata University,
Chiba, 273-0866,
Japan
sin_ei1@yahoo.co.jp
Abstract:
We introduce a generalized additivity of a mapping between Banach spaces and establish the Ulam type stability problem for a generalized additive mapping. The obtained results are somewhat different from the Ulam type stability result of Euler-Lagrange type mappings obtained by H. -M. Kim, K. -W. Jun and J. M. Rassias.
Paper's Title:
Some Distortion and Other Properties Associated with a Family of the n-Fold Symmetric Koebe Type Functions
Author(s):
H. M. Srivastava, N. Tuneski and E. Georgieva-Celakoska
Department of Mathematics and Statistics,
University of Victoria,
Victoria, British Columbia V8W 3R4,
Canada
Faculty of Mechanical Engineering, St.
Cyril and Methodius University,
Karpo'v s II b.b., MK-1000 Skopje,
Republic of Macedonia
Abstract:
In a recent work by Kamali and Srivastava [5], a certain family of the n-fold symmetric Koebe type functions was introduced and studied systematically. In an earlier investigation, Eguchi and Owa [4] had considered its special case when n=1 (see also [10]). Here, in our present sequel to these earlier works, this general family of the n-fold symmetric Koebe type functions is studied further and several distortion theorems and such other properties as the radii of spirallikeness, the radii of starlikeness and the radii of convexity, which are associated with this family of the n-fold symmetric Koebe type functions, are obtained. We also provide certain criteria that embed this family of the n-fold symmetric Koebe type functions in a function class Gλ which was introduced and studied earlier by Silverman [7].
Paper's Title:
On Reformations of 2--Hilbert Spaces
Author(s):
M. Eshaghi Gordji, A. Divandari, M. R. Safi and Y. J. Cho
Department of Mathematics, Semnan
University,
P.O. Box 35195--363, Semnan,
Iran
meshaghi@semnan.ac.ir, madjid.eshaghi@gmail.com
Department of Mathematics, Semnan
University,
Iran
Department of Mathematics, Semnan
University,
Iran
safi@semnan.ac.ir, SafiMohammadReza@yahoo.com
Department of Mathematics Education and
the RINS,
Gyeongsang National University
Chinju 660-701,
Korea
Abstract:
In this paper, first, we introduce the new concept of (complex) 2--Hilbert spaces, that is, we define the concept of 2--inner product spaces with a complex valued 2--inner product by using the 2--norm. Next, we prove some theorems on Schwartz's inequality, the polarization identity, the parallelogram laws and related important properties. Finally, we give some open problems related to 2--Hilbert spaces.
Paper's Title:
A Note on Divergent Fourier Series and λ-Permutations
Author(s):
A. Castillo, J. Chavez and H. Kim
Tufts University,
Department of Mathematics,
Medford, MA 02155,
USA
E-mail: angel.castillo@tufts.edu
Texas Tech University,
Department of Mathematics and Statistics,
Lubbock, TX 79409,
USA
E-mail: josechavez5@my.unt.edu
University of Michigan-Dearborn,
Department of Mathematics and Statistics,
Dearborn, MI 48128,
USA.
E-mail: khyejin@umich.edu
Abstract:
We present a continuous function on [-π,π] whose Fourier series diverges and it cannot be rearranged to converge by a λ-permutation.
Paper's Title:
The Functional Equation With an Exponential Polynomial Solution and its Stability
Author(s):
Young Whan Lee1, Gwang Hui Kim2*, and Jeong Il Kim3
1Department of Computer
Hacking and Information Security,
College of Engineering, Daejeon University,
Daejeon, 34520,
Korea(Republic of).
E-mail: ywlee@dju.ac.kr
2Department of Mathematics,
Kangnam University,
Yongin, Gyeonggi, 16979,
Korea(Republic of).
E-mail: ighkim@kangnam.ac.kr
3Department of Statistics,
Daejeon University,
Daejeon 34520,
Korea(Republic of).
E-mail: jlkim@dju.ac.kr
Abstract:
In this paper, we prove that the unique continuous solution of the functional equation

is

where pn(x) is a polynomial with degree n and

We also obtain the superstability and stability of the functional equation with the following forms, respectively:

and

Paper's Title:
Convergence Speed of Some Random Implicit-Kirk-type Iterations for Contractive-type Random Operators
Author(s):
H. Akewe, K.S. Eke
Department of Mathematics,
Covenant University,
Canaanland, KM 10, Idiroko Road, P. M. B. 1023, Ota, Ogun State,
Nigeria.
E-mail: hudson.akewe@covenantuniversity.edu.ng,
kanayo.eke@covenantuniversity.edu.ng
Abstract:
The main aim of this paper is to introduce a stochastic version of multistep type iterative scheme called a modified random implicit-Kirk multistep iterative scheme and prove strong convergence and stability results for a class of generalized contractive-type random operators. The rate of convergence of the random iterative schemes are also examined through an example. The results show that our new random implicit kirk multistep scheme perform better than other implicit iterative schemes in terms of convergence and thus have good potentials for further applications in equilibrium problems in computer science, physics and economics.
Paper's Title:
Several New Closed-form Evaluations of the Generalized Hypergeometric Function with Argument 1/16
Author(s):
B. R. Srivatsa Kumar, Insuk Kim and Arjun K. Rathie
Department of Mathematics,
Manipal Institute of Technology,
Manipal Academy of Higher Education,
Manipal 576 104,
India.
E-mail: sri_vatsabr@yahoo.com
Department of Mathematics Education,
Wonkwang University,
Iksan, 54538,
Republic of Korea.
E-mail: iki@wku.ac.kr
Department of Mathematics,
Vedant College of Engineering and Technology,
Rajasthan Technical University,
Bundi, 323021, Rajasthan,
India.
E-mail: arjunkumarrathie@gmail.com
Abstract:
The main objective of this paper is to establish as many as thirty new closed-form evaluations of the generalized hypergeometric function q+1Fq(z) for q= 2, 3, 4. This is achieved by means of separating the generalized hypergeometric function q+1Fq(z) for q=1, 2, 3, 4, 5 into even and odd components together with the use of several known infinite series involving central binomial coefficients obtained earlier by Ji and Hei \& Ji and Zhang.
Paper's Title:
Dyadic Riesz Wavelets on Local Fields of Positive Characteristics
Author(s):
Kartik Garg, Raj Kumar, Satyapriya
Department of Mathematics,
University of Delhi,
Delhi,
India.
kartikgarg1421@gmail.com,
rajkmc@gmail.com
kmc.satyapriya@gmail.com
Abstract:
In this research paper, we introduce a novel theory for the construction of a Riesz wavelet basis in the space L2(K), where K is a local field with positive characteristics. Our approach is two fold: firstly, we derive some essential characterizations of the scaling function associated with the structure of a Riesz MRA on a local field, and secondly, we review existing methods for constructing wavelet frames in L2(K). We also present a well elaborated example for a better comprehension of our theory. Due to mathematical convenience, we limit ourselves to the case of dyadic dilations only.
Paper's Title:
On a Novel Class of Advanced Integrals of MacRobert Type Incorporating Generalized Hypergeometric Functions
Author(s):
Shwetha S Shetty and Shantha Kumari Kurumujji
Department of Mathematics, A J Institute
of Engineering and Technology,
(Affiliated to Visvesvaraya Technological University (VTU), Belagavi),
Mangaluru, Karnataka,
India.
E-mail: shwethashetty576@gmail.com,
shanthakk99@gmail.com
Abstract:
The main objective of this study is to evaluate two master formulas expressed as integrals over the interval from zero to π/2. These integrals involve exponential functions and trigonometric functions sine and cosine, along with 3F2 hypergeometric functions parameterized by general variables i and j, where i = j = 0, ± 1, ± 2, .... From these master formulas, we derive twenty-six MacRobert-type integrals by evaluating them at specific values of i and j. The results are expressed using Gamma functions. The findings given here are derived from summation formulas for the series 4 F3 obtained recently by Choi and Rathie along with a general result attributed to MacRobert.
Paper's Title:
The successive approximations method and error estimation in terms of at most the first derivative for delay ordinary differential equations
Author(s):
Alexandru Mihai Bica
Department of Mathematics,
University of Oradea,
Str. Armatei Romane no.5,
410087, Oradea,
Romania
smbica@yahoo.com
abica@uoradea.ro
Abstract:
We present here a numerical method for first order delay ordinary differential
equations, which use the Banach's fixed point theorem, the sequence of
successive approximations and the trapezoidal quadrature rule. The error
estimation of the method uses a recent result of P. Cerone and S.S. Dragomir
about the remainder of the trapezoidal quadrature rule for Lipchitzian
functions and for functions with continuous first derivative.
Paper's Title:
Logarithmically complete monotonicity properties for the gamma functions
Author(s):
Chao-Ping Chen and Feng Qi
Department of Applied Mathematics and Informatics,
Research Institute of Applied Mathematics,
Henan Polytechnic University,
Jiaozuo City, Henan 454010,
China.
chenchaoping@hpu.edu.cn
Department of Applied Mathematics and Informatics,
Research Institute of Applied Mathematics,
Henan Polytechnic University,
Jiaozuo City, Henan 454010,
China.
qifeng@hpu.edu.cn
fengqi618@member.ams.org
Abstract:
Some logarithmically completely monotonic functions involving the gamma functions are presented. As a consequence, some known results are proved and refined.
Paper's Title:
On Sandwich Theorems for Certain Subclass of Analytic Functions Involving Dziok-Srivastava Operator
Author(s):
T. N. Shanmugam, M. P. Jeyarama and A. Singaravelu
Department of Mathematics
College of Engineering, Anna University
Chennai - 600 025,
India
drtns2001@yahoo.com
Department of Mathematics
Easwari Engineering College
Ramapuram, Chennai - 600089
Tamilnadu, India
jeyaraman-mp@yahoo.co.i
Department of Mathematics
Valliammai Engineering College
Chennai - 603203
Tamilnadu, India.
asing-59@yahoo.com
Abstract:
The purpose of this present paper is to derive some subordination and superordination results for certain normalized analytic functions in the open unit disk, acted upon by Dziok-Srivastava operator. Relevant connections of the results, which are presented in this paper, with various known results are also considered.
Paper's Title:
Some Inequalities for a Certain Class of Multivalent Functions
Using Multiplier Transformation
Author(s):
K. Suchithra, B. Adolf Stephen, A. Gangadharan and S. Sivasubramanian
Department Of Applied Mathematics
Sri Venkateswara College Of Engineering
Sriperumbudur, Chennai - 602105,
India.
suchithravenkat@yahoo.co.in
Department Of Mathematics,
Madras Christian College
Chennai - 600059,
India.
adolfmcc2003@yahoo.co.in
Department Of Applied Mathematics
Sri Venkateswara College Of Engineering
Sriperumbudur, Chennai - 602105,
India.
ganga@svce.ac.in
Department Of Mathematics,
Easwari Engineering College
Ramapuram, Chennai - 600089,
India.
ganga@svce.ac.in
Abstract:
The object of the present paper is to derive several inequalities
associated with differential subordinations between analytic functions
and a linear operator defined for a certain family of p-valent
functions, which is introduced here by means of a family of extended
multiplier transformations. Some special cases and consequences of
the main results are also considered.
Paper's Title:
On Stan Ulam and his Mathematics
Author(s):
Krzysztof Ciesielski and Themistocles M. Rassias
Mathematics Institute, Jagiellonian University,
Abstract:
In this note we give a glimpse of the curriculum vitae of Stan Ulam, his personality and some of the mathematics he was involved in.
Paper's Title:
Applications of Relations and Relators in the
Extensions of Stability Theorems for Homogeneous and Additive Functions
Author(s):
Árpád Száz
Institute of Mathematics, University of Debrecen,
H-4010 Debrecen,
Pf. 12,
Hungary
szaz@math.klte.hu
Abstract:
By working out an appropriate technique of relations and relators and extending the ideas of the direct methods of Z. Gajda and R. Ger, we prove some generalizations of the stability theorems of D. H. Hyers, T. Aoki, Th. M. Rassias and P. Găvruţă in terms of the existence and unicity of 2-homogeneous and additive approximate selections of generalized subadditive relations of semigroups to vector relator spaces. Thus, we obtain generalizations not only of the selection theorems of Z. Gajda and R. Ger, but also those of the present author.
Paper's Title:
Ulam Stability of Reciprocal Difference and Adjoint Functional Equations
Author(s):
K. Ravi, J. M. Rassias and B. V. Senthil Kumar
Department of Mathematics,
Sacred Heart College, Tirupattur - 635601,
India
Pedagogical Department E. E.,
Section of Mathematics and Informatics,
National and Capodistrian University of Athens,
4, Agamemnonos Str., Aghia Paraskevi,
Athens, Attikis 15342,
GREECE
Department of Mathematics,
C.Abdul Hakeem College of Engineering and
Technology, Melvisharam - 632 509, India
shckavi@yahoo.co.in
jrassias@primedu.uoa.gr
bvssree@yahoo.co.in
Abstract:
In this paper, the reciprocal difference functional equation (or RDF equation) and the reciprocal adjoint functional equation (or RAF equation) are introduced. Then the pertinent Ulam stability problem for these functional equations is solved, together with the extended Ulam (or Rassias) stability problem and the generalized Ulam (or Ulam-Gavruta-Rassias) stability problem for the same equations.
Paper's Title:
Subordination Results Associated with Hadamard Product
Author(s):
S. Sivasubramanian, C. Ramachandran and B. A. Frasin
Department of Mathematics,
University College of Engineering,
Anna University,
Saram-604 307,
India
Department of Mathematics,
University College of Engineering,
Anna University,
Villupuram,
India
Department of Mathematics,
Al al-Bayt University,
P.O. Box: 130095 Mafraq,
Jordan
Abstract:
In the present investigation, we consider an unified class of functions of complex order using Hadamard's convolution. We obtain a necessary and sufficient condition for functions to be in these classes.
Paper's Title:
On the Three Variable Reciprocity Theorem and Its Applications
Author(s):
D. D. Somashekara and D. Mamta
Department of Studies in Mathematics,
University of Mysore,
Manasagangotri, Mysore-570 006
India
dsomashekara@yahoo.com
Department of Mathematics,
The National Institute of Engineering,
Mysore-570 008,
India
mathsmamta@yahoo.com
Abstract:
In this paper we show how the three variable reciprocity theorem can be easily derived from the well known two variable reciprocity theorem of Ramanujan by parameter augmentation. Further we derive some q-gamma, q-beta and eta-function identities from the three variable reciprocity theorem.
Paper's Title:
Applications of the Structure Theorem of (w1,w2)-Tempered
Ultradistributions
Author(s):
Hamed M. Obiedat and Lloyd E. Moyo
Department of Mathematics,
Hashemite University,
P.O.Box 150459, Zarqa13115,
Jordan.
E-mail: hobiedat@hu.edu.j
Department of Mathematics, Computer
Science & Statistics,
Henderson State University,
1100 Henderson Street, Arkadelphia, AR 71999,
USA.
E-mail: moyol@hsu.edu
Abstract:
Using a previously obtained structure theorem for (w1, w2)-tempered ultradistributions, we prove that these ultradistributions can be represented as initial values of solutions of the heat equation.
Paper's Title:
Inequalities for Discrete F-Divergence Measures: A Survey of Recent Results
Author(s):
Sever S. Dragomir1,2
1Mathematics, School of Engineering
& Science
Victoria University, PO Box 14428
Melbourne City, MC 8001,
Australia
E-mail: sever.dragomir@vu.edu.au
2DST-NRF Centre of Excellence in the Mathematical and Statistical Sciences,
School of Computer Science & Applied Mathematics,
University of the Witwatersrand,
Private Bag 3, Johannesburg 2050,
South Africa
URL:
http://rgmia.org/dragomir
Abstract:
In this paper we survey some recent results obtained by the author in providing various bounds for the celebrated f-divergence measure for various classes of functions f. Several techniques including inequalities of Jensen and Slater types for convex functions are employed. Bounds in terms of Kullback-Leibler Distance, Hellinger Discrimination and Varation distance are provided. Approximations of the f-divergence measure by the use of the celebrated Ostrowski and Trapezoid inequalities are obtained. More accurate approximation formulae that make use of Taylor's expansion with integral remainder are also surveyed. A comprehensive list of recent papers by several authors related this important concept in information theory is also included as an appendix to the main text.
Paper's Title:
On Commutator of Aluthge Transforms and Fuglede-Putnam Property
Author(s):
(Manzar Maleki, Ali Reza Janfada and Seyed Mohammad Sadegh Nabavi Sales
International Campus, Faculty of
Mathematical Sciences,
Ferdowsi University of Mashhad, Mashhad,
Iran.
E-mail: manzar.maleki@gmail.com
Faculty of Mathematics and Statistics,
Department of Mathematics,
University of Birjand,
P. O. Box 414, Birjand 9717851367,
Iran.
E-mail: ajanfada@birjand.ac.ir
Department of Pure Mathematics, Hakim
Sabzevari University,
P.O. Box 397, Sabzevar,
Iran.
E-mail: sadegh.nabavi@hsu.ac.ir
Abstract:
We deal with the well-known Fuglede-Putnam theorem and related FP-property. We show that if
(A,B) has the FP-property, then so has
![]() where 0≤
t1,t2≤1 are arbitrary.
We first prove that
where 0≤
t1,t2≤1 are arbitrary.
We first prove that
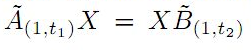 if and only if
AX=XB for all X, whenever (A,B) has the FP-property. We
prove some similar results for
if and only if
AX=XB for all X, whenever (A,B) has the FP-property. We
prove some similar results for
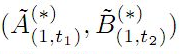 instead of $
instead of $![]() as well. Also we introduce the sequence of generalized iterations of Aluthge transform of operators and express some results for this notion associated to the FP-property.
as well. Also we introduce the sequence of generalized iterations of Aluthge transform of operators and express some results for this notion associated to the FP-property.
Paper's Title:
On Euler's First Transformation Formula for k-hypergeometric Function
Author(s):
Sungtae Jun and Insuk Kim
General Education Institute,
Konkuk University, Chungju 380-701,
Republic of Korea.
E-mail: sjun@kku.ac.kr
Department of Mathematics Education,
Wonkwang University, Iksan, 570-749,
Republic of Korea.
E-mail: iki@wku.ac.kr
Abstract:
Mubeen et al. obtained Kummer's first transformation for the k-hypergeometric function. The aim of this note is to provide the Euler-type first transformation for the k-hypergeometric function. As a limiting case, we recover the results of Mubeen et al. In addition to this, an alternate and easy derivation of Kummer's first transformation for the k-hypergeometric function is also given.
Paper's Title:
Trace Inequalities for Operators in Hilbert Spaces: a Survey of Recent Results
Author(s):
Sever S. Dragomir1,2
1Mathematics,
School of Engineering
& Science
Victoria University,
PO Box 14428
Melbourne City, MC 8001,
Australia
E-mail: sever.dragomir@vu.edu.au
2DST-NRF Centre of Excellence in the Mathematical and Statistical Sciences,
School of Computer Science & Applied Mathematics,
University of the Witwatersrand,
Private Bag 3, Johannesburg 2050,
South Africa
URL:
https://rgmia.org/dragomir
Abstract:
In this paper we survey some recent trace inequalities for operators in Hilbert spaces that are connected to Schwarz's, Buzano's and Kato's inequalities and the reverses of Schwarz inequality known in the literature as Cassels' inequality and Shisha-Mond's inequality. Applications for some functionals that are naturally associated to some of these inequalities and for functions of operators defined by power series are given. Further, various trace inequalities for convex functions are presented including refinements of Jensen inequality and several reverses of Jensen's inequality. Hermite-Hadamard type inequalities and the trace version of Slater's inequality are given. Some Lipschitz type inequalities are also surveyed. Examples for fundamental functions such as the power, logarithmic, resolvent and exponential functions are provided as well.
Paper's Title:
Estimation for Bounded Solutions of Some Nonlinear Integral Inequalities with Delay in Several Variables
Author(s):
Smakdji Mohamed Elhadi, Denche Mouhamed and Khellaf Hassane
Department of Mathematics
University of Frères Mentouri
PO Box 25000, Ain Elbay,
Constantine,
Algeria.
E-mail: khellafhassane@umc.edu.dz
Abstract:
In this paper, some new nonlinear retarded integral inequalities of Gronwall-Bellman type for functions of two and n-independents variables are investigated. The derived results can be applied in the study of differential-integral equations with time delay. An example is given to illustrate the application of our results.
Paper's Title:
Differential Equations for Indicatrices, Spacelike and Timelike Curves
Author(s):
Sameer, Pradeep Kumar Pandey
Department of Mathematics,
Jaypee University of Information Technology,
Solan, Himachal Pradesh,
India.
E-mail: sksameer08@gmail.com,
pandeypkdelhi@gmail.com
Abstract:
Motivated by the recent work of Deshmukh et al. [20], in this paper we show that Tangent, Binormal, and Principal Normal indicatrices do not form non-trivial differential equations. Finally, we obtain the 4th-order differential equations for spacelike and timelike curves.
Paper's Title:
Using Direct and Fixed Point Technique of Cubic Functional Equation and its Hyers-Ulam Stability
Author(s):
Ramanuja Rao Kotti, Rajnesh Krishnan Mudaliar, Kaushal Neelam Devi, Shailendra Vikash Narayan
Fiji National University,
Department of Mathematics & Statistics,
P.O. Box 5529, Lautoka,
Fiji.
E-mail: ramanuja.kotti@fnu.ac.fj
URL: https://www.fnu.ac.fj
Abstract:
In this present work, we introduce a new type of finite dimensional cubic functional equation of the form

where Φ≥4 is an integer, and derive its general solution. The main purpose of this work is to investigate the Hyers-Ulam stability results for the above mentioned functional equation in Fuzzy Banach spaces by means of direct and fixed point methods.
Paper's Title:
Some New Inequalities for Hypo-q-Norms on a Cartesian Product of Normed Linear Spaces
Author(s):
Sever S. Dragomir1,2
1Applied
Mathematics Research Group, ISILC,
Victoria University,
PO Box 14428, Melbourne City, MC 8001,
Australia.
E-mail: sever.dragomir@vu.edu.au
URL:
http://rgmia.org/dragomir
2School of Computer Science & Applied Mathematics,
University of the Witwatersrand,
Private Bag 3, Johannesburg 2050,
South Africa.
Abstract:
Let ( E,|| · ||) be a normed
linear space over the real or complex number field K. If by
Sn,p with p∈[ 1,∞]
we denote the spheres generated by the $p$-norms ||
· ||n,p on Kn, then we consider
the following hypo-q-norms on
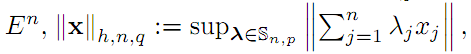 with q>1 and
with q>1 and
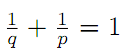 if p>1, q=1 if
p=∞
and q=∞ if p=1. For p=2, we also consider the hypo-Euclidean norm on En, i.e.,
if p>1, q=1 if
p=∞
and q=∞ if p=1. For p=2, we also consider the hypo-Euclidean norm on En, i.e.,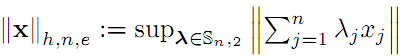
In this paper we have obtained among others the following inequalities
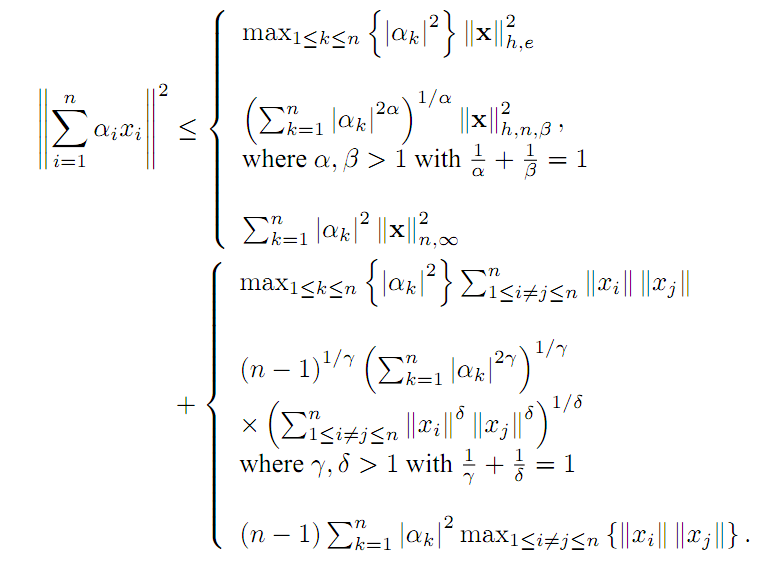
The case for n=2 and the connection with the following new norms
![]() and
and
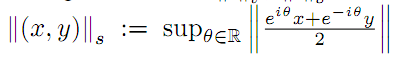
are also investigated. When the norm || · || is generated by an inner product, further bounds are given as well.
Paper's Title:
On Certain Classes of Harmonic Univalent Functions Based on Salagean Operator
Author(s):
G. Murugusundaramoorthy, Thomas Rosy, and B. A. Stephen
Department of Applied Mathematics and Informatics,
Department of Mathematics, Vellore Institute of Technology,
Deemed University, Vellore - 632014, India.
gmsmoorthy@yahoo.com
Department of Applied Mathematics and Informatics,
Department of Mathematics, Madras Christian College,
Chennai - 600059, India.
drthomasrosy@rediffmail.com
Abstract:
We define and investigate a class of complex-valued harmonic univalent functions of the form f = h + g using Salagean operator where h and g are analytic in the unit disc U = { z : |z| < 1 }. A necessary and sufficient coefficient conditions are given for functions in these classes. Furthermore, distortion theorems, inclusion relations, extreme points, convolution conditions and convex combinations for this family of harmonic functions are obtained.
Paper's Title:
Refinement Inequalities Among Symmetric Divergence Measures
Author(s):
Inder Jeet Taneja
Departamento de Matemática,
Universidade Federal de Santa Catarina, 88.040-900
Florianópolis, Sc, Brazil
taneja@mtm.ufsc.br
URL: http://www.mtm.ufsc.br/~taneja
Abstract:
There are three classical divergence measures in the literature
on information theory and statistics, namely, Jeffryes-Kullback-Leiber’s
J-divergence, Sibson-Burbea-Rao’s Jensen- Shannon divegernce and
Taneja’s arithemtic - geometric mean divergence. These bear an
interesting relationship among each other and are based on logarithmic
expressions. The divergence measures like Hellinger discrimination,
symmetric χ2−divergence, and triangular discrimination
are not based on logarithmic expressions. These six divergence measures are
symmetric with respect to probability distributions. In this paper some
interesting inequalities among these symmetric divergence measures are studied.
Refinements of these inequalities are also given. Some inequalities due to
Dragomir et al. [6]
are also improved.
Paper's Title:
Iterative Approximation of Common Fixed Points of a Finite Family of Asymptotically Hemi-contractive Type Mappings
Author(s):
Jui-Chi Huang
Center for General Education,
Northern Taiwan Institute of Science and Technology,
Peito, Taipei,
Taiwan, 11202, R.O.C.
juichi@ntist.edu.tw
Abstract:
In this paper, we prove that the sequence of
the modified Ishikawa-Xu,
Ishikawa-Liu, Mann-Xu and Mann-Liu iterative types of a finite family of
asymptotically hemi-contractive type mappings converges strongly to a common
fixed point of the family in a real p-uniformly convex Banach space with
p>1. Our results improve and extend some recent results.
Paper's Title:
Generalizations of Hermite-Hadamard's Inequalities for Log-Convex Functions
Author(s):
Ai-Jun Li
School of Mathematics and Informatics,
Henan Polytechnic University,
Jiaozuo City, Henan Province,
454010, China.
liaijun72@163.com
Abstract:
In this article, Hermite-Hadamard's inequalities are extended in
terms of the weighted power mean and log-convex
function. Several refinements, generalizations and related
inequalities are obtained.
Paper's Title:
On a Method of Proving the Hyers-Ulam Stability
of Functional Equations on Restricted Domains
Author(s):
Janusz Brzdęk
Department of Mathematics
Pedagogical University Podchor
Abstract:
We show that generalizations of some (classical) results on the Hyers-Ulam stability of functional equations, in several variables, can be very easily derived from a simple result on stability of a functional equation in single variable
Paper's Title:
Harmonic Functions with Positive Real Part
Author(s):
Sïbel Yalçin
Uludag Universitesi,
Fen Edebiyat Fakultesi, Matematik Bolumu,
16059 Bursa,
Turkey
Abstract:
In this paper, the class of harmonic functions f=h+{g} with positive real part and normalized by f(ζ )=1, (|ζ|<1) is studied, where h and g are analytic in U={z:|z|<1}. Some properties of this class are searched. Sharp coefficient relations are given for functions in this class. On the other hand, the author make use of Alexander integral transforms of certain analytic functions (which are starlike with respect to f(ζ)) with a view to investigating the construction of sense preserving, univalent and close to convex harmonic functions.
Paper's Title:
Some Identities for Ramanujan - Göllnitz - Gordon Continued fraction
Author(s):
M. S. Mahadeva Naika, B. N. Dharmendra and S. Chandan Kumar
Department of Mathematics,
Bangalore University,
Central College Campus,
Bangalore-560 001,
INDIA
Department of Mathematics,
Maharani's Science College for Women,
J. L. B. Road, Mysore-570 001,
INDIA
Abstract:
In this paper, we obtain certain P--Q eta--function identities, using which we establish identities providing modular relations between Ramanujan-Göllnitz-Gordon continued fraction H(q) and H(q^n) for n= 2, 3, 4, 5, 7, 8, 9, 11, 13, 15, 17, 19, 23, 25, 29 and 55.
Paper's Title:
Hyponormal and K-Quasi-Hyponormal Operators On Semi-Hilbertian Spaces
Author(s):
Ould Ahmed Mahmoud Sid Ahmed and Abdelkader Benali
Mathematics Department,
College of Science,
Aljouf University,
Aljouf 2014,
Saudi Arabia.
E-mail:
sididahmed@ju.edu.sa
Mathematics Department, Faculty of
Science,
Hassiba Benbouali, University of Chlef,
B.P. 151 Hay Essalem, Chlef 02000,
Algeria.
E-mail:
benali4848@gmail.com
Abstract:
Let H be a Hilbert space and let A be a positive bounded operator on H. The semi-inner product < u|v>A:=<Au|v>, u,v ∈ H induces a semi-norm || .||A on H. This makes H into a semi-Hilbertian space. In this paper we introduce the notions of hyponormalities and k-quasi-hyponormalities for operators on semi Hilbertian space (H,||.||A), based on the works that studied normal, isometry, unitary and partial isometries operators in these spaces. Also, we generalize some results which are already known for hyponormal and quasi-hyponormal operators. An operator T ∈ BA (H) is said to be (A, k)-quasi-hyponormal if
![]()
Paper's Title:
Robust Error Analysis of Solutions to Nonlinear Volterra Integral Equation in Lp Spaces
Author(s):
Hamid Baghani, Javad Farokhi-Ostad and Omid Baghani
Department of Mathematics, Faculty of
Mathematics,
University of Sistan and Baluchestan, P.O. Box 98135-674, Zahedan,
Iran.
E-mail:
h.baghani@gmail.com
Department of Mathematics, Faculty of
Basic Sciences,
Birjand University of Technology, Birjand,
Iran.
E-mail: j.farrokhi@birjandut.ac.ir
Department of Mathematics and Computer
Sciences,
Hakim Sabzevari University, P.O. Box 397, Sabzevar,
Iran.
E-mail:
o.baghani@gmail.com
Abstract:
In this paper, we propose a novel strategy for proving an important inequality for a contraction integral equations. The obtained inequality allows us to express our iterative algorithm using a "for loop" rather than a "while loop". The main tool used in this paper is the fixed point theorem in the Lebesgue space. Also, a numerical example shows the efficiency and the accuracy of the proposed scheme.
Paper's Title:
The Dynamics of an Ebola Epidemic Model with Quarantine of Infectives
Author(s):
Eliab Horub Kweyunga
Department of Mathematics,
Kabale University,
P.O.Box 317, Kabale,
Uganda.
E-mail: hkweyunga@kab.ac.ug
Abstract:
The recurrent outbreaks of ebola in Africa present global health challenges. Ebola is a severe, very fatal disease with case fatality rates of up to 90%. In this paper, a theoretical deterministic model for ebola epidemic with quarantine of infectives is proposed and analyzed. The model exhibits two equilibria; the disease free and endemic equilibrium points. The basic reproduction number, R0, which is the main threshold, is obtained and the stability of the equilibrium points established. Using parameter values drawn from the 2014 West Africa ebola outbreak, a numerical simulation of the model is carried out. It is found that the dynamics of the model are completely determined by R0 and that a quarantine success rate of at least 70% is sufficient to contain the disease outbreak.
Paper's Title:
Some fixed point results in partial S-metric spaces
Author(s):
M. M. Rezaee, S. Sedghi, A. Mukheimer, K. Abodayeh, and Z. D. Mitrovic
Department of Mathematics, Qaemshahr
Branch,
Islamic Azad University, Qaemshahr,
Iran.
E-mail: Rezaee.mohammad.m@gmail.com
Department of Mathematics, Qaemshahr
Branch,
Islamic Azad University, Qaemshahr,
Iran.
E-mail: sedghi.gh@qaemiau.ac.ir
Department of Mathematics and General
Sciences,
Prince Sultan University, Riyadh,
KSA.
E-mail: mukheimer@psu.edu.sa
Department of Mathematics and General
Sciences,
Prince Sultan University, Riyadh,
KSA.
E-mail: kamal@psu.edu.sa
Nonlinear Analysis Research Group,
Faculty of Mathematics and Statistics,
Ton Duc Thang University, Ho Chi Minh City,
Vietnam.
E-mail: zoran.mitrovic@tdtu.edu.vn
Abstract:
We introduce in this article a new class of generalized metric spaces, called partial S-metric spaces. In addition, we also give some interesting results on fixed points in the partial S-metric spaces and some applications.
Paper's Title:
ψ(m,q)-Isometric Mappings on Metric Spaces
Author(s):
Sid Ahmed Ould Beinane, Sidi Hamidou Jah and Sid Ahmed Ould Ahmed Mahmoud
Mathematical Analysis and Applications,
Mathematics Department, College of Science,
Jouf University,
Sakaka P.O.Box 2014,
Saudi Arabia.
E-mail: beinane06@gmail.com
Department of Mathematics, College of
Science Qassim University,
P.O. Box 6640, Buraydah 51452,
Saudi Arabia.
E-mail: jahsiidi@yahoo.fr
Mathematical Analysis and Applications,
Mathematics Department, College of Science, Jouf University,
Sakaka P.O.Box 2014,
Saudi Arabia.
E-mail: sidahmed@ju.edu.sa,
sidahmed.sidha@gmail.com
Abstract:
The concept of (m,p)-isometric operators on Banach space was extended to
(m,q)-isometric mappings on general metric spaces in [6].
This paper is devoted to define the concept of
ψ(m, q)-isometric, which is the
extension of A(m, p)-isometric operators on Banach spaces introduced in [10].
Let T,ψ: (E,d) -> (E, d) be two mappings.
For some positive integer m and q ∈ (0,∞).
T is said to be an ψ(m,q)-isometry,
if for all y,z ∈ E,

Paper's Title:
Sharp Inequalities Between Hölder and Stolarsky Means of Two Positive Numbers
Author(s):
M. Bustos Gonzalez and A. I. Stan
The University of Iowa,
Department of Mathematics,
14 MacLean Hall,
Iowa City, Iowa,
USA.
E-mail:
margarita-bustosgonzalez@uiowa.edu
The Ohio State University at Marion,
Department of Mathematics,
1465 Mount Vernon Avenue,
Marion, Ohio,
USA.
E-mail: stan.7@osu.edu
Abstract:
Given any index of the Stolarsky means, we find the greatest and least indexes of the H\"older means, such that for any two positive numbers, the Stolarsky mean with the given index is bounded from below and above by the Hölder means with those indexes, of the two positive numbers. Finally, we present a geometric application of this inequality involving the Fermat-Torricelli point of a triangle.
Paper's Title:
Generalized Von Neumann-Jordan Constant for Morrey Spaces and Small Morrey Spaces
Author(s):
H. Rahman and H. Gunawan
Department of Mathematics,
Islamic State University Maulana Malik Ibrahim Malang,
Jalan Gajayana No.50,
Indonesia.
E-mail: hairur@mat.uin-malang.ac.id
Analysis and Geometry Group,
Faculty of Mathematics and Natural Sciences,
Bandung Institute of Technology, Bandung 40132,
Indonesia.
E-mail: hgunawan@math.itb.ac.id
URL:
http://personal.fmipa.itb.ac.id/hgunawan/
Abstract:
In this paper we calculate some geometric constants for Morrey spaces and small Morrey spaces, namely generalized Von Neumann-Jordan constant, modified Von Neumann-Jordan constants, and Zbaganu constant. All these constants measure the uniformly nonsquareness of the spaces. We obtain that their values are the same as the value of Von Neumann-Jordan constant for Morrey spaces and small Morrey spaces.
Paper's Title:
Algorithms for Nonlinear Problems Involving Strictly Pseudocontractive Mappings
Author(s):
Mathew Olajiire Aibinu1, Surendra Colin Thakur2, Sibusiso Moyo3
1Institute for Systems Science
& KZN E-Skill CoLab,
Durban University of Technology,
Durban 4000,
South Africa.
1DSI-NRF
Centre of Excellence in Mathematical and Statistical Sciences (CoE-MaSS),
Johannesburg,
South Africa.
E-mail: moaibinu@yahoo.com
mathewa@dut.ac.za
2 KZN E-Skill CoLab,
Durban University of Technology,
Durban 4000,
South Africa.
E-mail: thakur@dut.ac.za
3Institute for Systems Science & Office of the DVC Research,
Innovation & Engagement Milena Court,
Durban University of Technology,
Durban 4000,
South Africa.
E-mail: dvcrie@dut.ac.za
Abstract:
The puzzles in approximating a fixed point of nonlinear problems involving the class of strictly pseudocontractive mappings are conquered in this paper through viscosity implicit rules. Using generalized contraction mappings, a new viscosity iterative algorithm which is implicit in nature is proposed and analysed in Banach spaces for the class of strictly pseudocontractive mappings. The computations and analysis which are used in the proposed scheme are easy to follow and this gives rooms for a broad application of the scheme. It is obtained that the proposed iterative algorithm converges strongly to a fixed point of a μ-strictly pseudocontractive mapping which also solves a variational inequality problem. The result is also shown to hold for finite family of strictly pseudocontractive mappings. A numerical example is given to show the skillfulness of the proposed scheme and its implementation.
Paper's Title:
SQIRV Model for Omicron Variant with Time Delay
Author(s):
S. Dickson, S. Padmasekaran, G. E. Chatzarakis and S. L. Panetsos
Mathematics, Periyar University, Periyar
Palkalai Nagar, Salem,
636011, Tamilnadu,
India.
E-mail:
dickson@periyaruniversity.ac.in,
padmasekarans@periyaruniversity.ac.in
Electrical and Electronic Engineering
Educators, School of
Pedagogical and Technological Education (ASPETE),
Marousi 15122, Athens,
Greece.
E-mail: geaxatz@otenet.gr,
spanetsos@aspete.gr
Abstract:
In order to examine the dynamics of the Omicron variant, this paper uses mathematical modelling and analysis of a SQIRV model, taking into account the delay in the conversion of susceptible individuals into infected individuals and infected individuals into recovered individuals. The pandemic was eventually controlled as a result of the massive delays. To assure the safety of the host population, this concept incorporates quarantine and the COVID-19 vaccine. Both local and global stability of the model are examined. It is found that the fundamental reproduction number affects both local and global stability conditions. Our findings show that asymptomatic cases caused by an affected population play an important role in increasing Omicron infection in the general population. The most recent data on the pandemic Omicron variant from Tamil Nadu, India, is verified.
Paper's Title:
New Refinements for Integral and Sum Forms of Generalized Hölder Inequality For N Term
Author(s):
M. Jakfar, Manuharawati, D. Savitri
Mathematics Department,
Universitas Negeri Surabaya
Jalan Ketintang Gedung C8, Surabaya, 60321
Indonesia.
E-mail: muhammadjakfar@unesa.ac.id
manuharawati@unesa.ac.id
diansavitri@unesa.ac.id
Abstract:
We know that in the field of functional analysis, Hölder inequality is very well known, important, and very applicable. So many researchers are interested in discussing these inequalities. Many world mathematicians try to improve these inequalities. In general, the Hölder inequality has two forms, namely the integral form and the sum form. In this paper, we will introduce a new refinement of the generalization of Hölder inequalities in both integral and addition forms. Especially in the sum form, improvements will be introduced that are better than the previous improvements that have been published by Jing-feng Tian, Ming-hu Ha, and Chao Wang.
Paper's Title:
On the Class of Totally Polynomially Posinormal Operators
Author(s):
E. Shine Lal, T. Prasad, P. Ramya
Department of Mathematics,University
College,
Thiruvananthapuram, Kerala, 695034.
India.
E-mail: shinelal.e@gmail.com
Department of Mathematics,
University of Calicut,
Malapuram, Kerala 673635,
India.
E-mail: prasadvalapil@gmail.com
Department of Mathematics,
N.S.S College,
Nemmara, Kerala, 678508
India.
E-mail: ramyagcc@gmail.com
Abstract:
In this paper, we proved that if T ∈
B(H) is totally P-posinormal operator with
 . Moreover, we study spectral continuity and range kernel orthogonality of these class of operators.
. Moreover, we study spectral continuity and range kernel orthogonality of these class of operators.
Paper's Title:
Corrigendum for Differential Equations for Indicatrices, Spacelike and Timelike Curves
Author(s):
Sameer, Pradeep Kumar Pandey
Department of Mathematics,
Jaypee University of Information Technology,
Solan, Himachal Pradesh,
India.
E-mail: sksameer08@gmail.com,
pandeypkdelhi@gmail.com
ABSTRACT NOT FOUND. WEBSITE ERROR
Abstract:
Paper's Title:
On the Oscillatory Behavior of Self Adjoint Fractional Extensible Beam Equations
Author(s):
S. Priyadharshini1, G.E. Chatzarakis2, S. L. Panetsos2 and V. Sadhasivam1
1Post
Graduate and Research Department of Mathematics,
Thiruvalluvar Government Arts College,
Rasipuram - 637 401, Namakkal Dt., Tamil Nadu,
India.
E-mail: s.priya25april@gmail.com,
ovsadha@gmail.com
2Department
of Electrical and Electronic Engineering Educators,
School of Pedagogical and Technological Education(ASPETE),
Marousi 15122, Athens,
Greece.
E-mail: geaxatz@otenet.gr,
gea.xatz@aspete.gr,
spanetsos@aspete.gr
Abstract:
The main objective of this paper is to study the oscillatory behavior of the solutions of self adjoint fractional extensible beam equations by using integral average method. Some new sufficient conditions are established with various boundary conditions over a cylindrical domains. Examples illustrating the results are given.
Paper's Title:
On the Existence of Solutions for Functional Integral Inclusions
Author(s):
Y. Khouni and M. Denche
Faculty of Nature and Life Sciences,
University of Batna 2,
53 Road of constantine,
Fesdis,
Batna - 05078,
Algeria.
E-mail: y.khouni@univ-batna2.dz
Laboratoire Equations Differentielles,
Departement de Mathematiques, Faculte des Sciences Exactes,
Universite Freres Mentouri, Constantine 1,
25000 Constantine,
Algeria.
E-mail: m.denche@umc.edu.dz
Abstract:
In this paper, we study the sufficient conditions for the existence of compact sets of solutions for a class of functional integral inclusions, that are based on a fixed point theorem of Dhage, we also give the existence of integrable solutions by using the nonlinear alternative of Leray-Schauder. An illustrative examples and applications are given in the end of this paper.
Search and serve lasted 0 second(s).