Department of Mathematics, North Dakota State University,
Fargo, ND 58105,
USA
Abraham.Ungar@ndsu.edu
URL: http://math.ndsu.nodak.edu/faculty/ungar/

Department of Mathematics and Statistics,
York University, 4700 Keele St, Toronto,
ON M3J 1P3,
Canada.
E-mail: jmaxwellcampbell@gmail.com

Department of Mathematics, University of Taipei,
  No. 1, Ai-Guo West Road,
Taipei 10048, Taiwan.
E-mail: kwchen@uTaipei.edu.tw
URL: https://math.utaipei.edu.tw/p/412-1082-22.php

Abstract:

The modified Abel lemma on summation by parts has been applied in many ways recently to determine closed-form evaluations for infinite series involving generalized harmonic numbers with an upper parameter of two. We build upon such results using an integration technique that we apply to ``convert'' a given evaluation for such a series into an evaluation for a corresponding series involving squared harmonic numbers.

Paper's Title:

SQIRV Model for Omicron Variant with Time Delay

Author(s):

S. Dickson, S. Padmasekaran, G. E. Chatzarakis and S. L. Panetsos

Mathematics, Periyar University, Periyar Palkalai Nagar, Salem,
636011, Tamilnadu,
India.
E-mail: dickson@periyaruniversity.ac.in, padmasekarans@periyaruniversity.ac.in

Electrical and Electronic Engineering Educators, School of
Pedagogical and Technological Education (ASPETE),
Marousi 15122, Athens,
Greece.
E-mail: geaxatz@otenet.gr, spanetsos@aspete.gr

Abstract:

In order to examine the dynamics of the Omicron variant, this paper uses mathematical modelling and analysis of a SQIRV model, taking into account the delay in the conversion of susceptible individuals into infected individuals and infected individuals into recovered individuals. The pandemic was eventually controlled as a result of the massive delays. To assure the safety of the host population, this concept incorporates quarantine and the COVID-19 vaccine. Both local and global stability of the model are examined. It is found that the fundamental reproduction number affects both local and global stability conditions. Our findings show that asymptomatic cases caused by an affected population play an important role in increasing Omicron infection in the general population. The most recent data on the pandemic Omicron variant from Tamil Nadu, India, is verified.

Paper's Title:

Using Direct and Fixed Point Technique of Cubic Functional Equation and its Hyers-Ulam Stability

Author(s):

Ramanuja Rao Kotti, Rajnesh Krishnan Mudaliar, Kaushal Neelam Devi, Shailendra Vikash Narayan

Fiji National University,
Department of Mathematics & Statistics,
P.O. Box 5529, Lautoka,
Fiji.
E-mail: ramanuja.kotti@fnu.ac.fj
URL: https://www.fnu.ac.fj

Abstract:

In this present work, we introduce a new type of finite dimensional cubic functional equation of the form

where Φ≥4 is an integer, and derive its general solution. The main purpose of this work is to investigate the Hyers-Ulam stability results for the above mentioned functional equation in Fuzzy Banach spaces by means of direct and fixed point methods.

Paper's Title:

New Proofs of the Grüss Inequality

Author(s):

A.Mc.D. Mercer and Peter R. Mercer

Abstract:

We present new proofs of the Grüss inequality in its original form and in its linear functional form.

Paper's Title:

Refinement Inequalities Among Symmetric Divergence Measures

Author(s):

Inder Jeet Taneja

Departamento de Matemática,
Universidade Federal de Santa Catarina, 88.040-900
Florianópolis, Sc, Brazil
taneja@mtm.ufsc.br
URL: http://www.mtm.ufsc.br/~taneja

Abstract:

There are three classical divergence measures in the literature on information theory and statistics, namely, Jeffryes-Kullback-Leiber’s J-divergence, Sibson-Burbea-Rao’s Jensen- Shannon divegernce and Taneja’s arithemtic - geometric mean divergence. These bear an interesting relationship among each other and are based on logarithmic expressions. The divergence measures like Hellinger discrimination, symmetric χ²−divergence, and triangular discrimination are not based on logarithmic expressions. These six divergence measures are symmetric with respect to probability distributions. In this paper some interesting inequalities among these symmetric divergence measures are studied. Refinements of these inequalities are also given. Some inequalities due to Dragomir et al. [6] are also improved.

Paper's Title:

Oscillations of First Order Linear Delay Difference Equations

Author(s):

G. E. Chatzarakis and I. P. Stavroulakis

Department of Mathematics, University of Ioannina,
451 10, Greece
ipstav@cc.uoi.gr

Abstract:

Consider the first order linear delay difference equation of the form   where  is a sequence of nonnegative real numbers, k is a positive integer and  denotes the forward difference operator  New oscillation criteria are established when the well-known oscillation conditions  and  are not satisfied. The results obtained essentially improve known results in the literature.

Paper's Title:

Weighted Generalization of the Trapezoidal Rule via Fink Identity

Author(s):

S. Kovač, J. Pečarić and A. Vukelić

Faculty of Geotechnical Engineering, University of Zagreb,
Hallerova aleja 7, 42000 Varaždin,
Croatia.
sanja.kovac@gtfvz.hr

Faculty of Textile Technology, University of Zagreb,
Pierottijeva 6, 10000 Zagreb,
Croatia.
pecaric@hazu.hr

Faculty of Food Technology and Biotechnology, Mathematics department, University of Zagreb,
Pierottijeva 6, 10000 Zagreb,
Croatia.
avukelic@pbf.hr

Abstract:

The weighted Fink identity is given and used to obtain generalized weighted trapezoidal formula for n-time differentiable functions. Also, an error estimate is obtained for this formula.

Paper's Title:

Oscillation and Boundedness of Solutions to First and Second Order Forced Dynamic Equations with Mixed Nonlinearities

Author(s):

Ravi P. Agarwal and Martin Bohner

Department of Mathematical Sciences, Florida Institute of Technology
Melbourne, FL 32901,
U.S.A.
bohner@mst.edu
URL:http://web.mst.edu/~bohner

Department of Economics and Finance, Missouri University of Science and Technology
Rolla, MO 65401,
U.S.A.
agarwal@fit.edu

Abstract:

Some oscillation and boundedness criteria for solutions to certain first and second order forced dynamic equations with mixed nonlinearities are established. The main tool in the proofs is an inequality due to Hardy, Littlewood and Pólya. The obtained results can be applied to differential equations, difference equations and q-difference equations. The results are illustrated with numerous examples.

Paper's Title:

Integer Sums of Powers of Trigonometric Functions (MOD p), for prime p

Author(s):

G. J. Tee

Department of Mathematics, University of Auckland, Auckland,
New Zealand
tee@math.auckland.ac.nz

Abstract:

Many multi--parameter families of congruences (mod p) are found for integer sums of  q^th powers of the trigonometric functions over various sets of equidistant arguments, where p is any prime factor of q. Those congruences provide sensitive tests for the accuracy of software for evaluating trigonometric functions to high precision.

Paper's Title:

Positive Periodic Solutions for Second-Order Differential Equations with Generalized Neutral Operator

Author(s):

Wing-Sum Cheung, Jingli Ren and Weiwei Han

Abstract:

By some analysis of the neutral operator and an application of the fixed-point index theorem, we obtain sufficient conditions for the existence, multiplicity and nonexistence of periodic solutions to a second-order differential equation with the prescribed neutral operator, which improve and extend some recent results of Lu-Ge, Wu-Wang, and Zhang. An example is given to illustrate our results. Moreover, the analysis of the generalized neutral operator will be helpful for other types of differential equations.

Paper's Title:

Shape Diagrams for 2D Compact Sets - Part I: Analytic Convex Sets.

Author(s):

S. Rivollier, J. Debayle and J.-C. Pinoli

Ecole Nationale Supérieure des Mines de Saint-Etienne,

CIS - LPMG, UMR CNRS 5148, 158 cours Fauriel,

42023 Saint-Etienne Cedex 2, France.

 rivollier@emse.fr; debayle@emse.fr; pinoli@emse.fr
 

Abstract:

Shape diagrams are representations in the Euclidean plane introduced to study 3-dimensional and 2-dimensional compact convex sets. Such a set is represented by a point within a shape diagram whose coordinates are morphometrical functionals defined as normalized ratios of geometrical functionals. Classically, the geometrical functionals are the area, the perimeter, the radii of the inscribed and circumscribed circles, and the minimum and maximum Feret diameters. They allow thirty-one shape diagrams to be built. Most of these shape diagrams can also been applied to more general compact sets than compact convex sets. Starting from these six classical geometrical functionals, a detailed comparative study has been performed in order to analyze the representation relevance and discrimination power of these thirty-one shape diagrams. The purpose of this paper is to present the first part of this study, by focusing on analytic compact convex sets. A set will be called analytic if its boundary is piecewise defined by explicit functions in such a way that the six geometrical functionals can be straightforwardly calculated. The second and third part of the comparative study are published in two following papers [19.20]. They are focused on analytic simply connected sets and convexity discrimination for analytic and discretized simply connected sets, respectively.

Paper's Title:

On the Degree of Approximation of Continuous Functions that Pertains to the Sequence-To-Sequence Transformation

Author(s):

Xhevat Z. Krasniqi

University of Prishtina,
Department of Mathematics and Computer Sciences,
5 Mother Teresa Avenue, Prishtinë, 10000,
Republic of Kosovo.
 

xheki00@hotmail.com

Abstract:

In this paper we prove analogous theorems like Leindler's 3 using the so-called A-transform of the B-transform of the partial sums of Fourier series. In addition, more than two such transforms are introduced and for them analogous results are showed as well.

Paper's Title:

On an Elliptic Over-Determined Problem in Dimension Two

Author(s):

Lakhdar Ragoub
Department of Mathematics and Information of Tiyadhechnology
AL Yamamah University
P.O. Box 45 180, Riyadh 11 512
Saudi Arabia.

lragoub@yu.edu.sa

Abstract:

We extend the method of Weinberger for a non-linear over-determined elliptic problem
in R². We prove that the domain in consideration is a ball. The tool of this investigation are maximum principles and P-functions.

Paper's Title:

Some Double λ-Convergent Sequence Spaces Over n-Normed Spaces

Author(s):

Kuldip Raj, Renu Anand and Seema Jamwal

School of Mathematics,
Shri Mata Vaishno Devi University Katra-182320,
Jammu and Kashmir,
India.

E-mail: kuldipraj68@gmail.com,  renuanand71@gmail.com, seemajamwal8@gmail.com

Abstract:

In this paper we introduce some double generalized λ-convergent sequence spaces over n-normed spaces defined by Musielak-Orlicz function M = (M_k,l). We also made an attempt to study some topological and algebraic properties of these sequence spaces.

Paper's Title:

On Singular Numbers of Hankel Matrices of Markov Functions

Author(s):

Vasily A. Prokhorov

Department of Mathematics and Statistics,
University of South Alabama,
Mobile, Alabama 36688-0002,
USA.
E-mail: prokhoro@southalabama.edu
URL: http://www.southalabama.edu/mathstat/people/prokhorov.shtml

Abstract:

Let E ⊂ (01,1) be a compact set and let μ be a positive Borel measure with support supp μ=E. Let

In the case when E=[a,b]⊂ (-1,1) and μ satisfies the condition dμ/dx>0 a.e. on E, we investigate asymptotic behavior of singular numbers σ_kn,n of the Hankel matrix D_n, where k_n/n→θ∈[0,1] as n→∞. Moreover, we obtain asymptotics of the Kolmogorov, Gelfand and linear k-widths, k=k_n, of the unit ball A_n,2 of P_n∩L₂(Γ) in the space L₂(μ,E), where Γ={z:|z|=1} and P_n is the class of all polynomials of the degree at most n.

Paper's Title:

On Interpolation of L² functions

Author(s):

Anis Rezgui

Department of Mathematics,
Faculty of Sciences,
Taibah University, Al Madina Al Munawara,
KSA.

Mathematics Department,
INSAT,
University of Carthage, Tunis,
Tunisia
E-mail: anis.rezguii@gmail.com

Abstract:

In this paper we are interested in polynomial interpolation of irregular functions namely those elements of L²(R,μ) for μ a given probability measure. This is of course doesn't make any sense unless for L² functions that, at least, admit a continuous version. To characterize those functions we have, first, constructed, in an abstract fashion, a chain of Sobolev like subspaces of a given Hilbert space H₀. Then we have proved that the chain of Sobolev like subspaces controls the existence of a continuous version for L² functions and gives a pointwise polynomial approximation with a quite accurate error estimation.

Paper's Title:

Applications of the Structure Theorem of (w₁,w₂)-Tempered Ultradistributions

Author(s):

Hamed M. Obiedat and Lloyd E. Moyo

Department of Mathematics,
Hashemite University,
P.O.Box 150459, Zarqa13115,
Jordan.
E-mail: hobiedat@hu.edu.j

Department of Mathematics, Computer Science & Statistics,
Henderson State University,
1100 Henderson Street, Arkadelphia, AR 71999,
USA.
E-mail: moyol@hsu.edu

Abstract:

Using a previously obtained structure theorem for (w₁, w₂)-tempered ultradistributions, we prove that these ultradistributions can be represented as initial values of solutions of the heat equation.

Paper's Title:

Existence of Positive Solutions for Nonlinear Fractional Differential Equations with Multi-point Boundary Conditions

Author(s):

N. Adjeroud

Khenchela University, Department of Mathematics,
Khenchela, 40000,
Algeria.
E-mail: adjnac@gmail.com

Abstract:

This paper is devoted to the existence results of positive solutions for a nonlinear fractional differential equations with multi-point boundary conditions. By means of the Schauder fixed point theorem, some results on the existence are obtained.

Paper's Title:

Introducing the Dorfmanian: A Powerful Tool for the Calculus Of Variations

Author(s):

Olivier de La Grandville

Department of Management Science and Engineering,
Stanford University,
475 Via Ortega, Stanford, CA 94305,
U. S. A.

E-mail: odelagrandville@gmail.com

Abstract:

We show how a modified Hamiltonian proposed by Robert Dorfman [1] to give intuitive sense to the Pontryagin maximum principle can be extended to easily obtain all high-order equations of the calculus of variations. This new concept is particularly efficient to determine the differential equations leading to the extremals of functionals defined by n-uple integrals, while a traditional approach would require -- in some cases repeatedly -- an extension of Green's theorem to n-space.
Our paper is dedicated to the memory of Robert Dorfman (1916 - 2002).

Paper's Title:

A Low Order Least-Squares Nonconforming Finite Element Method for Steady Magnetohydrodynamic Equations

Author(s):

Z. Yu, D. Shi and H. Zhu

College of Science,
Zhongyuan University of Technology,
Zhengzhou 450007,
China.
E-mail: 5772@zut.edu.cn

School of Mathematics and Statistics,
Zhengzhou University,
Zhengzhou 450001,
China.
E-mail: shi_dy@126.com

Mathematics Department,
University of Southern Mississippi,
Hattiesburg MS, 39406,
U.S.A
E-mail: huiqing.zhu@usm.edu

Abstract:

A low order least-squares nonconforming finite element (NFE) method is proposed for magnetohydrodynamic equations with EQ₁^rot element and zero-order Raviart-Thomas element. Based on the above element's typical interpolations properties, the existence and uniqueness of the approximate solutions are proved and the optimal order error estimates for the corresponding variables are derived.

Paper's Title:

Bounds on the Jensen Gap, and Implications for Mean-Concentrated Distributions

Author(s):

Xiang Gao, Meera Sitharam, Adrian E. Roitberg

Department of Chemistry, and Department of Computer & Information Science & Engineering,
University of Florida,
Gainesville, FL 32611,
USA.
E-mail: qasdfgtyuiop@gmail.com
URL: https://scholar.google.com/citations?user=t2nOdxQAAAAJ

Abstract:

This paper gives upper and lower bounds on the gap in Jensen's inequality, i.e., the difference between the expected value of a function of a random variable and the value of the function at the expected value of the random variable. The bounds depend only on growth properties of the function and specific moments of the random variable. The bounds are particularly useful for distributions that are concentrated around the mean, a commonly occurring scenario such as the average of i.i.d. samples and in statistical mechanics.

Paper's Title:

Structural and Spectral Properties of k-Quasi Class Q Operators

Author(s):

Valdete Rexhëbeqaj Hamiti and Shqipe Lohaj

Department of Mathematics,
Faculty of Electrical and Computer Engineering,
University of Prishtina "Hasan Prishtina",
Prishtine 10000,
Kosova.
E-mail: valdete.rexhebeqaj@uni-pr.edu

Department of Mathematics,
Faculty of Electrical and Computer Engineering,
University of Prishtina "Hasan Prishtina",
Prishtine 10000,
Kosova.
E-mail: shqipe.lohaj@uni-pr.edu

Abstract:

An operator is said to be k-quasi class Q if , for all where k is a natural number. In this paper, first we will prove some results for the matrix representation of k-quasi class Q operators. Then, we will give the inclusion of approximate point spectrum of k-quasi class Q operators. Also, we will give the equivalence between Aluthge transformation and *-Aluthge transformation of k-quasi class Q operators.

Paper's Title:

Solving Non-Autonomous Nonlinear Systems of Ordinary Differential Equations Using Multi-Stage Differential Transform Method

Author(s):

K. A. Ahmad, Z. Zainuddin, F. A. Abdullah

School of Mathematical Sciences Universiti Sains Malaysia
11800 USM Penang
Malaysia.
E-mail: abumohmmadkh@hotmail.com
zarita@usm.my
farahaini@usm.my

Abstract:

Differential equations are basic tools to describe a wide variety of phenomena in nature such as, electrostatics, physics, chemistry, economics, etc. In this paper, a technique is developed to solve nonlinear and linear systems of ordinary differential equations based on the standard Differential Transform Method (DTM) and Multi-stage Differential Transform Method (MsDTM). Comparative numerical results that we are obtained by MsDTM and Runge-Kutta method are proposed. The numerical results showed that the MsDTM gives more accurate approximation as compared to the Runge-Kutta numerical method for the solutions of nonlinear and linear systems of ordinary differential equations

Paper's Title:

Coexisting Attractors and Bubbling Route to Chaos in Modified Coupled Duffing Oscillators

Author(s):

B. Deruni¹, A. S. Hacinliyan^1,2, E. Kandiran³, A. C. Keles², S. Kaouache⁴, M.-S. Abdelouahab⁴, N.-E. Hamri⁴

¹Department of Physics,
University of Yeditepe,
Turkey.

²Department of Information Systems and Technologies,
University of Yeditepe,
Turkey

³Department of Software Development,
University of Yeditepe,
Turkey.

⁴Laboratory of Mathematics and their interactions,
University Center of Abdelhafid Boussouf,
Mila 43000,
Algeria.

E-mail: berc890@gmail.com
ahacinliyan@yeditepe.edu.tr
engin.kandiran@yeditepe.edu.tr
cihan.keles@yeditepe.edu.tr
s.kaouache@centr-univ-mila.dz
medsalah3@yahoo.fr
n.hamri@centre-univ-mila.dz

Abstract:

In this article dynamical behavior of coupled Duffing oscillators is analyzed under a small modification. The oscillators have cubic damping instead of linear one. Although single duffing oscillator has complex dynamics, coupled duffing systems possess a much more complex structure. The dynamical behavior of the system is investigated both numerically and analytically. Numerical results indicate that the system has double scroll attractor with suitable parameter values. On the other hand, bifurcation diagrams illustrate rich behavior of the system, and it is seen that, system enters into chaos with different routes. Beside classical bifurcations, bubbling route to chaos is observed for suitable parameter settings. On the other hand, Multistability of the system is indicated with the coexisting attractors, such that under same parameter setting the system shows different periodic and chaotic attractors. Moreover, chaotic synchronization of coupled oscillators is illustrated in final section.

Paper's Title:

Oscillatory Behavior of Second-Order Non-Canonical Retarded Difference Equations

Author(s):

G.E. Chatzarakis¹, N. Indrajith², E. Thandapani³ and K.S. Vidhyaa⁴

¹Department of Electrical and Electronic Engineering Educators,
School of Pedagogical and Technological Education,
Marousi 15122, Athens,
Greece.
E-mail:  gea.xatz@aspete.gr, geaxatz@otenet.gr
 

²Department of Mathematics,
Presidency College, Chennai - 600 005,
India.
E-mail: indrajithna@gmail.com

³Ramanujan Institute for Advanced Study in Mathematics,
University of Madras,
Chennai - 600 005,
India.
E-mail: ethandapani@yahoo.co.in

⁴Department of Mathematics,
SRM Easwari Engineering College,
Chennai-600089,
India.
E-mail: vidyacertain@gmail.com

Abstract:

Using monotonic properties of nonoscillatory solutions, we obtain new oscillatory criteria for the second-order non-canonical difference equation with retarded argument

Our oscillation results improve and extend the earlier ones. Examples illustrating the results are provided.

Paper's Title:

On Trigonometric Approximation of Continuous Functions by Deferred Matrix Means

Author(s):

Xhevat Zahir Krasniqi

Faculty of Education,
University of Prishtina "Hasan Prishtina",
Avenue "Mother Theresa " no. 5, Prishtinë 10000,
Republic of Kosovo.
E-mail: xhevat.krasniqi@uni-pr.edu
URL: https://staff.uni-pr.edu/profile/xhevatkrasniqi

Abstract:

In this paper, for the first time, we introduce the deferred matrix means which contain the well-known generalized deferred Nörlund, deferred Nörlund, deferred Riesz, deferred Cesàro means introduced earlier by others, and a new class of sequences (predominantly a wider class than the class of Head Bounded Variation Sequences). In addition, using the deferred matrix means of Fourier series of a continuous function, we determine the degree of approximation of such function via its modulus of continuity and a positive mediate function.

Paper's Title:

Improved Oscillation Criteria of Second-Order Advanced Non-canonical Difference Equation

Author(s):

G. E. Chatzarakis¹, N. Indrajith², S. L. Panetsos¹, E. Thandapani³

¹Department of Electrical and Electronic Engineering Educators
School of Pedagogical and Technological Education,
Marousi 15122, Athens,
Greece.
E-mail: gea.xatz@aspete.gr, geaxatz@otenet.gr
spanetsos@aspete.gr

²Department of Mathematics,
Presidency College, Chennai - 600 005,
India.
E-mail: indrajithna@gmail.com

³Ramanujan Institute for Advanced Study in Mathematics,
University of Madras Chennai - 600 005,
India.
E-mail: ethandapani@yahoo.co.in

Abstract:

Employing monotonic properties of nonoscillatory solutions, we derive some new oscillation criteria for the second-order advanced non-canonical difference equation

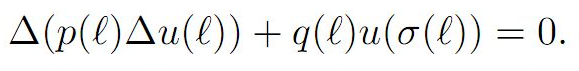
Our results extend and improve the earlier ones. The outcome is illustrated via some particular difference equations.

Paper's Title:

Walrasian Equilibrium for Set-valued Mapping

Author(s):

M. Muslikh, R.B.E Wibowo, S. Fitri

Department of Mathematics,
University of Brawijaya,
Malang,
Indonesia.
E-mail: mslk@ub.ac.id
rbagus@ub.ac.id
saadatul@ub.ac.id

Abstract:

In this article, we obtain the existence of Walras equilibrium for set-valued demand mappings in a pure exchange economy. In this case, the set-valued mappings are defined by the loss function. Therefore, we shall summarize the features describing the exchange economy system which contain the loss function.

Paper's Title:

ℵ0 Algebra and its Novel Application in Edge Detection

Author(s):

G. E. Chatzarakis¹, S. Dickson², S. Padmasekaran², S. L. Panetsos¹, and J. Ravi³

¹Electrical and Electronic Engineering Educators,
School of Pedagogical and Technological Education (ASPETE),
Marousi 15122, Athens,
Greece.
E-mail: geaxatz@otenet.gr, spanetsos@aspete.gr

²Mathematics, Periyar University,
Periyar Palkalai Nagar, Salem, 636011, Tamilnadu,
India.
E-mail: dix.bern@gmail.com, padmasekarans@periyaruniversity.ac.in

³Department of Mathematics, Amity University,
Bengaluru, Karnataka,
India.
E-mail: jravistat@gmail.com
 

Abstract:

In this paper a new type of ℵ₀-algebra has been defined. With its help, the fuzzy cross subalgebra and the fuzzy η-relation on the ℵ₀-algebra are introduced and their respective properties are derived. Moreover, the fuzzy cross ℵ₀-ideal of the ℵ₀-algebra is defined with some theorems and intuitionistic fuzzy ℵ₀-ideals of the ℵ₀-algebra are introduced. This fuzzy algebra concept is applied in image processing to detect edges. This ℵ₀-algebra is a novelty in the field of research.

Paper's Title:

Solving Fixed Point Problems and Variational Inclusions Using Viscosity Approximations

Author(s):

P. Patel, R. Shukla

Department of Mathematics, School of Advanced Sciences, VIT-AP University\\
Amaravati, 522237, Andhra Pradesh,
India.
E-mail: prashant.patel9999@gmail.com, prashant.p@vitap.ac.in

Department of Mathematical Sciences & Computing, Walter Sisulu University,
Mthatha 5117,
South Africa.
E-mail: rshukla@wsu.ac.za

Abstract:

This paper proposes a new algorithm to find a common element of the fixed point set of a finite family of demimetric mappings and the set of solutions of a general split variational inclusion problem in Hilbert spaces. The algorithm is based on the viscosity approximation method, which is a powerful tool for solving fixed point problems and variational inclusion problems. Under some conditions, we prove that the sequence generated by the algorithm converges strongly to this common solution.

Search and serve lasted 0 second(s).